Riemann–Roch theorem for algebraic curves

In algebraic geometry, the Riemann–Roch theorem is a central result for smooth and complete algebraic curves over algebraically closed fields.

Contents

Necessary notions

A curve X designates a smooth and complete algebraic variety of dimension 1 over an algebraically closed field 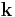\mathbf k .

A Weil divisor D on X is a finite linear combination with integer coefficients of points of X. Its index (or degree), ind D, is the sum of the coefficients. We denote by I(D) the dimension (necessarily finite) of the 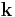\mathbf k vector space of rational functions on the curve (elements of the function field of that curve) whose divisor is greater than D.

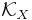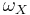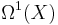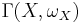The canonical divisor \mathcal K_X is the divisor associated to the canonical bundle \omega_X cotangent. In the case of a curve, the cotangent bundle is a line bundle that coincides with \Omega^1(X). The geometric genus g of the curve is the dimension of the space of global sections of the canonical bundle \Gamma(X, \omega_X).

Statement of the theorem

Let X be an algebraic curve of genus g. Then for any divisor D, one has

I(D) - I(\mathcal K_X - D) = \mathrm{ind} D %2B 1 - g. \,

History of the result

Obviously, the theorem originates in the works of Riemann on complex analytic curves, or Riemann surfaces, and more specifically in the classical Riemann-Roch theorem, whose statement and proof published in 1865 are due to Gustav Roch.

The first proof for general algebraic curves is due to F. K. Schmidt in 1931 as he was working on perfect fields of finite characteristic. Under the hand of Pierre Roquette:

The first main achievement of F. K. Schmidt is the discovery that the classical theorem of Riemann-Roch on compact Riemann surfaces can be transferred to function fields with finite base field. Actually, his proof of the Riemann-Roch theorem works for arbitrary perfect base fields, not necessarily finite.

The theorem was later generalized in higher dimension by Hirzebruch in 1954 as the Hirzebruch-Riemann-Roch theorem and further in 1957 in a relative context by Alexander Grothendieck as the Grothendieck-Riemann-Roch theorem. Grothendieck introduced for the proof what came to be known as Grothendieck groups, which led to the foundation of K-theory and motivated the proof of the Atiyah-Singer index theorem (1963).

Some applications

An irreducible plane curve of degree d has (d-1)(d-2)/2-g singularities, when properly counted. It follows that, if a curve has (d-1)(d-2)/2 different singularities, it is a rational curve and, thus, admits a rational parameterization.

The theorem implies in particular that the canonical divisor has index 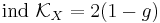\mathrm{ind}\ \mathcal K_X = 2 (1 - g ), the Euler characteristic of X.

This theorem can be thought of as an elementary weak instance of Serre duality.

A proof via Serre duality

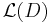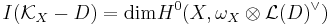The integer I(D) is the dimension of the space of global sections of the line bundle \mathcal L(D) associated to D (cf. Cartier divisor). In terms of sheaf cohomology, we therefore have I (D) = \mathrm {dim} H^0 (X, \mathcal L(D)), and likewise I (\mathcal K_X - D) = \mathrm {dim} H^0 (X, \omega_X \otimes \mathcal L(D)^\vee) .

But Serre duality for non-singular projective varieties in the particular case of a curve states that H^0 (X, \omega_X \otimes \mathcal L(D)^\vee) is isomorphic to the dual \simeq H^1 (X, \mathcal L (D))^\vee. The left hand side thus equals the Euler characteristic of the divisor D. When D = 0 , we find the Euler characteristic for the structure sheaf ie 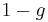1-g by definition.

To prove the theorem for general divisor, one can then proceed by adding points one by one to the divisor and taking some off and ensure that the Euler characteristic transforms accordingly to the right hand side.

See also

References